Objectives
The emergence of singularities, such as oscillations and concentrations, is at the heart of some of the most intriguing problems in the theory of nonlinear PDEs. Rich sources of these phenomena can be found for instance in the equations of mathematical material science and hyperbolic conservation laws.
The SINGULARITY project will investigate singularities through strategies that combine geometric measure theory with harmonic analysis.

Theme I investigates condensated singularities, i.e. singular parts of (vector) measures solving a PDE and the fine structure theory for PDE-constrained measures.
Theme II is concerned with the development of a compensated compactness theory for sequences of solutions to a PDE, which is capable of dealing with concentrations. The central aim is to study in detail the (non-)compactness properties of such sequences in the presence of asymptotic singularities.
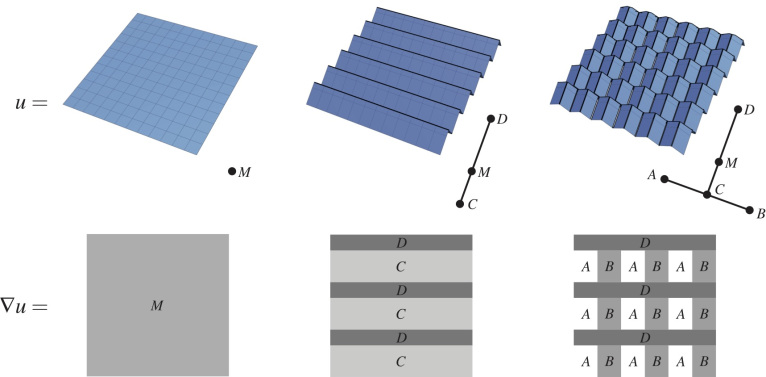
Theme III investigates higher-order microstructure, i.e. nested periodic oscillations in sequences, such as laminates. The main objective is to understand the effective propertiesof such microstructures.
We will also consider applications in Data Science, in particular in the detection & prediction of anomalies in streaming data. This sub-project is part of the Data-Centric Engineering (DCE) programme at the Alan Turing Institute.
A more technical description of recent results can be found in the following survey article: AfreeSurvey

