Andy Morris

I am a PhD research student investigating CP violation in the B system. My analysis involves the analysis of the decay $B^0 \to D_{C\!P} \pi^+ \pi^-$ which I am using to measure the angle $\beta$ in the Cabbibo-Kobyashi-Maskawa (CKM) unitary triangle^[1]. This investigation is being carried out at the LHCb experiment, at CERN.

As well as this, I am completing service work for the LHCb, helping to model the Velo upgrade for use in Monte Carlo simulations of results taken in LHC Run 3 (beginning 2021, probably delayed to 2022 due to Covid-19). This modelled geometry will be included as part of the $\textsc{Geant4}$ simulation of the LHCb detector. This is being completed both with the old DDDB description (composed of pure xml) as well as new DD4hep description (composed of xml and C++ together).

Description of my Analysis

CP violation in general describes the different behaviour of matter and anti-matter in certain interactions (in this case under the weak force); futhermore, any differences found in interactions after a CP operation is performed on a system will imply a fundamental difference between matter and anti-matter. The Standard Model of Particle Physics (the most cutting edge theory of particle physics which has been confirmed experimentally) models CP violation under the weak force using the (3 $\times$ 3) CKM matrix^[2]. A key point of this matrix is that it is unitary meaning it obeys:

$\sum_{i=1}^3 V_{ij}V^*_{ik} = \delta_{jk}$ (where $\delta_{jk}$ is the Kronecker delta).^[3]

Taking the six cases where this equation equals to zero, gives six possible times where the sum of three points gives zero. This implies six triangles in the complex plane, each of which give the full CKM description of CP violation.

The triangle which is most commonly used is described by this equation:

$V_{ud}V^*_{ub}+V_{cd}V^*_{cb}+V_{td}V^*_{tb} = 0$ .

The internal angles of this triangle are labelled $\alpha$ , $\beta$ and $\gamma$ . The measurement of angle $\beta$ is the intended outcome of this analysis. A schematic drawing of the unitarity triangle is given below:

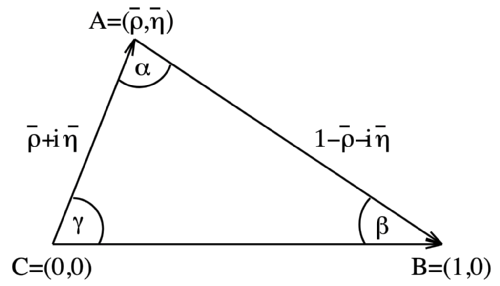
Schematic Drawing of CKM unitarity triangle. η and ρ are parameters from a representation of the CKM matrix (Wolfenstein parameters).

Schematic Drawing of CKM unitarity triangle. $\bar{\eta}$ and $\bar{\rho}$ are parameters from a representation of the CKM matrix (Wolfenstein parameters). Taken from [4].

The angle $\beta$ itself generally is not measured; rather, $\sin{2\beta}$ and $\cos{2\beta}$ are measured. The cutting edge measurements of $\sin{2\beta}$ are found from analyses of $B^0 \to J/\psi K^0_S$ decays (known as the 'golden mode' for this measurement). Using these decays and similar, $\sin{2\beta}$ has been measured to be: 0.680 $\pm$ 0.025^[5]. Measurements of $\cos{2\beta}$ are currently measured far less precisely than for $\sin{2\beta}$ .

Using the datasets from LHCb in both Run 1 and Run 2, this analysis is expected to be able to measure $\sin{2\beta}$ to a similar precision as in $B^0 \to J/\psi K^0_S$ decays and find a world-leading measurement of $\cos{2\beta}$ .

Current Progess

The current progress of my analysis is that the data has been obtained from the LHCb (in a process known as nTupling), this has then had a selection applied to it. The idea of this selection is to remove background to the data whilst retaining as much signal as possible. This is done with a combination of methods including vetoes, neural networks and boosted decision trees which are trained using Monte-Carlo distributions.

The nTuples with selection have also had their reconstructed $B^{0}$ -mass distribution fit using a series of probability density functions (PDFs) which are fit component-wise to different Monte Carlo distributions before a combination of the PDFs are fit to the total data to describe the relative yields of each background.

VeLo modelling

Since the specifics of my work are internal to LHCb; until it's published I can't show much of the specifics of my work. However, here is a gif of an older description of a single module of the new VeLo description.

gif of a single module and its supports as it will appear in the upgraded VeLo (a slightly simplistic version compared to the most recent)

References

[1] T. Latham and T. Gershon, A method to measure cos(2β) using time-dependent Dalitz plot analysis of $B^0 \to D_{C\!P} \pi^+ \pi^-$ , J. Phys.G36(2009) 025006, arXiv:0809.0872.

[2] M. Kobayashi and T. Maskawa, CP-Violation in the renormalizable theory of weak interaction, Prog. Theor. Phys.49(1973) 652.

[3] I. I. Bigi and A. I. Sanda,CP violation, , [Camb. Monogr. Part. Phys. Nucl. Phys. Cosmol.9,1(2009)].

[4] The CKM matrix and the unitarity triangle. Workshop, CERN, Geneva, Switzerland, 13-16 Feb 2002:Proceedings, 2003. doi: 10.5170/CERN-2003-002-corr.

[5] Particle Data Group, M. Tanabashiet al.,Review of particle physics, Phys. Rev.D98(2018) 030001.

_{Page last edited: 28 May 2019.}

Contact details:

E: Andy.Morris@Warwick.ac.uk

Andy.M@cern.ch

A: LHCb Office, P449

Department of Physics
University of Warwick
Coventry
West Midlands, UK
CV4 7AL