When certain musical notes are played together, they produce a pleasing sound and the phenomenon is called harmony. Pythagoras is reputed to have discovered a mathematical basis for this. They say he was so impressed by this discovery that he leapt to the conclusion that ‘All is Number!’ This is possibly the best known conceptual principle put forward by the Pythagorean school, a religious and philosophical community and movement that prospered in the Mediterranean world from the 6th to the 4th century BCE.

What they might have meant by this cryptic phrase is still subject to much debate, but the intimate relationship between musical harmony and simple ratios is often regarded as a basic manifestation that led to the general theory, explains Professor Minhyong Kim, from Warwick’s Department of Mathematics.

According to the mathematician Nichomachus (60-120 CE), himself a numerical mystic, Pythagoras first observed the relationship between maths and music from the resonant clang of hammers of different weights as they struck iron. Much more likely is that they noticed the relationship between musical pitch and lengths of vibrating strings in instruments like the lyre. In any case, the mathematical nature of various oscillations seems to be key to understanding the Pythagorean maxim as well as all its descendants developed over the subsequent millennia.

The main ingredient of our own perception of pitch is the change in air pressure near the listening ear.

The vibrating membrane of a loudspeaker, for example, will create alternating bands of high and low pressure that move rapidly across the room. 'Middle A' on the piano corresponds to the high pressure fronts going at a rate of 440 per second. Because of its speed, we aren’t sensitive enough to detect directly the rise and fall, but experience it rather as a pitch. The higher the frequency - the number of fronts per second - measured in the unit Hertz (Hz), the higher the pitch.

Even if it’s a bit tedious, you might be interested by the formula for the basic frequency produced by a string of length L on your violin or guitar:

When looking at formulae like this, you should try to focus on one bit at a time, temporarily relegating the other complications to the background. For example, let’s look at the effect of the L, which would have been the main Pythagorean interest. According to this, when you halve the length of the string, the frequency doubles, since you are dividing by something only half as large. Try this out if you have guitar and see how the pitch rises. Conversely, when the string length doubles, the frequency will fall by a half.

You can still experiment even without an actual instrument. Try an online tone generator , a machine that produces sounds with specific frequencies. To compare efficiently, open several browser pages at once, so you can run different frequencies on each one. In terms of perception, a doubling of frequencies corresponds to a pitch one octave higher, and it’s interesting to note that in some sense, it ‘sounds the same’ as the lower note. Increasing the frequency by 3/2, say from 440 to 660, produces an interval of fifth degree, and playing the two together generates the simplest kind of harmony.

Now you can think a bit about T, the tension of the string. This is the force along the length of the string, which you may regulate using pegs on your instrument. According to the formula, when you increase the tension fourfold, the pitch will double. Don’t try this on an actual string since you will likely break it while trying to effect such a large increase. In any case, you will find it easier to use the formula in the converse direction. How do you know when the tension has increased fourfold? When the pitch rises by an octave.

The term d is the density of the string, the amount of material packed into any given portion. You may have noticed on stringed instruments that the sound of a thicker string is lower.

What makes certain sounds musical?

Eduard Hanslick (1825-1904), one of the most influential music critics of 19th century Europe had this to say:

"Should the babbling of the brook, the splash of ocean waves, the thunder of avalanches, the raging of a cyclone, not have been the occasion and model for human music? Did all of the whispering, whistling, crashing sounds have nothing to do with our musical life? We must in fact answer in the negative. All of these manifestations of nature are just noise and sound, that is, successive irregular vibrations of air. Very seldom, and then, only in isolated instances, does nature produce a tone, that is, a sound of a specific measurable height and depth."

The Encyclopaedia Britannica essentially agrees with Hanslick in defining a tone as ‘a sound that can be recognised by its regularity of vibration.’

From the vantage point of modern science, it is hard to agree that nature does not produce tones. It’s just that they tend to come in a mixture or a spectrum of frequency.

You can get a sense of how to take a sound apart into the frequencies that make it up using an online spectrum analyser . There are some preloaded sounds, but you can upload short audio files yourself and analyse their makeup.

Here is a siren, for example:

Music versus maths

Musicians will probably argue that a tone is not just made up by the regularity of vibrations, which one might identify with pitch, but also concerns duration, intensity (or loudness), and timbre. The notion of timbre is possibly the trickiest of these to quantify. Some people define it as the quality of sound that remains after you have taken the other three into account. For example, a violin and a flute playing notes with exactly the same duration, pitch, and loudness still sound quite different. A large part of this can still be accounted for by the spectrum because any given pitch is actually accompanied by many other pitches in frequencies that are multiples of the basic one. Usually much weaker than the basic frequency, nevertheless they manage to modulate the tone quality. They are called overtones and correspond to different vibrational modes.

A violin has a much richer spectrum of overtones than a flute, for example. You can check this as well with the spectrum analyser. This link( https://www.youtube.com/watch?v=cnH2ltfW48U) takes you to a nice video illustrating the modes.

We can get a snapshot of the variation of pressure near our ear using a graph. Here is a picture of a tone of frequency 2 Hz (too low to be audible):

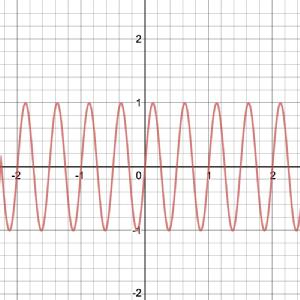
Image: Sound of 2 Hz

Moving from left to right represents the passage of time over which the height of the curve represents the rise and fall of pressure. The reason I showed you that slow inaudible note first is because something vaguely ‘normal’, say a frequency of 110, looks like this:

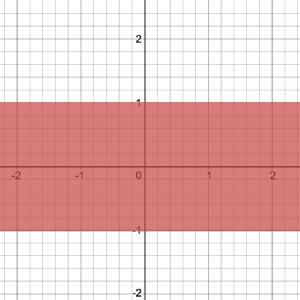
Image: Sound of 110 Hz

It’s just a solid band in this view, but by zooming in, you can see it resolve into rapid and sharp undulations.

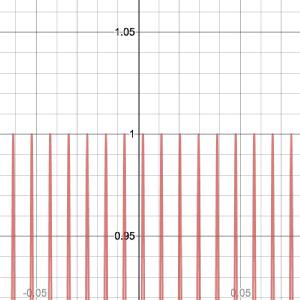
Image: Zooming in on the Sound of 110 Hz

Here is what happens when you mix the frequencies 2 and 20 in proportions of 10 to 1.

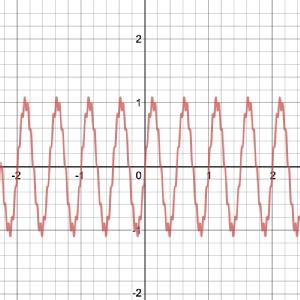
Image: 2 Hz+ (0.1)20 Hz

Remember, any tone you hear is actually a mixture, and this picture might give you a sense of how the quality is affected even while the significant frequency is retained. Finally, here is a picture of ‘white noise’, where a large number of frequencies are mixed in rather evenly.

Image: White noise: Frequency evenly distributed between 1 and 50 Hz

The sound of an ocean wave derided by Hanslick will tend to look like this. This idea that you can take apart sound (and light as well) into constituent frequencies, entities more fundamental than what is immediately audible, has had a profound impact on the development of science after the 19th century.

Importantly, the mathematical picture remains entirely analogous when we look into the microscopic details of nature following quantum mechanics. With only a little bit of exaggeration, one can say that the particles that make up the universe and the way they are put together resemble very much the fundamental frequencies combining into everyday sound and noise. So, from this point of view, the Pythagoreans may have been a good deal more right in their philosophy of nature than they could possibly have imagined.

In his recent book Absolute Music, the musicologist Mark Evan Bonds has written:

“The figures of Orpheus and Pythagoras embody two fundamentally different perspectives on music that together circumscribe the foundation of Western attitudes toward the art. As a musician, Orpheus demonstrated music’s effect; as a philosopher, Pythagoras explained its essence. Until at least the sixteenth century, and in many cases beyond, Pythagoras was credited with having discovered music’s essence–what it is–while Orpheus was hailed as the paradigmatic musician who demonstrated music’s effect–what it does.”

So even though we understand what music is physically, coming to a mathematical understanding of what music does even at the most elementary level, for example, why putting certain frequencies together is pleasing to the human senses, remains a task for the future.

 

Published:

16 June 2020

About:

Minhyong Kim, is the Christopher Zeeman Professor of Algebra, Geometry, and Public Understanding of Mathematics at Warwick Mathematics Institute.

Terms for republishing
The text in this article is licensed under a Creative Commons Attribution 4.0 International License (CC BY 4.0).

 

Share