Applied Mathematics Seminars
Organisers: Ferran Brosa Planella and Thomasina Ball
The Applied Maths Seminars are held on Fridays 12:00-13.00. This year the seminar will be hybrid (at least for Term 1): you can choose to attend in person in room B3.02 or on MS Teams. The team for the seminar is the same as last year, but if you are not a member, you can send a membership request via MS Teams or email the organisers.
Please contact Ferran Brosa Planella or Thomasina Ball if you have any speaker suggestions for future terms.
Seminar Etiquette: Here is a set of basic rules for the seminar.
- Please keep your microphone muted throughout the talk. If you want to ask a question, please raise your hand and the seminar organiser will (a) ask you to unmute if you are attending remotely or (b) get the speaker's attention and invite you to ask your question if you are in the room.
- If you are in the room with us, the room microphones capture anything you say very easily, and this is worth keeping in mind ☺️.
- You can choose to keep your camera on or not. Colleagues in the room will be able to see the online audience.
- Please let us know if you would like to meet and/or have lunch with any of the speakers who are coming to visit us so that I can make sure you have a place in the room.
Term 3
| Week | Date | Speaker | Online/F2F | Title |
| 1 | 28 Apr | Abdessamad Belfakir (Nottingham) | F2F | Estimating and Predicting Dynamics of Quantum Systems Using Probability Distribution Function and Klauder Coherent States (abstract) |
| 2 | 5 May | Hanne Kekkonen (TU Delft) | F2F | Random Tree Besov Priors (abstract) |
| 3 | 12 May | Luis Espath (Nottingham) | F2F | A continuum framework for phase field with bulk-surface dynamics (abstract) |
| 4 | 19 May | Rachel Bennett (Bristol) | F2F | Analytical framework for coordination of metachronal cilia (abstract) |
| 5 | 26 May | Thomas Barker (Cardiff) | F2F | Continuum modelling of granular flow with dynamic compressibility (abstract) |
| 6 | 2 Jun |
Jingbang Liu (Warwick) Ioana Bouros (Warwick) |
F2F |
Modelling rare rupture of nanoscale liquid thin films (abstract) Warwick-Lancaster global COVID-19 model (abstract) |
| 7 | 9 Jun | Masha Dvoriashyna (Oxford) | F2F | Bacterial hydrodynamics: reorientation during tumbles and viscoelastic lift (abstract) |
| 8 | 16 Jun | Edward Hinton (Melbourne) | Online | Containment of viscous fluid atop a crystal bed, and application to magmatic ore deposits (abstract) |
| 9 | 23 Jun | Susanne Horn (Coventry) | F2F | The Elbert Range of Turbulent Rotating Magnetoconvection (abstract) |
| 10 | 30 Jun | Eric Rogers (Southampton) POSTPONED | F2F |
Optimization-based Iterative Learning Control with Applications in Physics, Engineering, and Healthcare (abstract) |
Term 2
| Week | Date | Speaker | Online/F2F | Title |
| 1 | 13 Jan | Fabian Spill (Birmingham) | F2F | Mechanics, Geometry and Topology of Health and Disease (abstract) |
| 2 | 20 Jan | Bryn Davies (Imperial) | F2F | Are quasicrystals the future of metamaterial waveguides? (abstract) |
| 3 | 27 Jan | Graham Benham (Oxford) | F2F | From Olympic rowing to gunwale bobbing: Wave drag and wave thrust phenomena (abstract) |
| 4 | 3 Feb |
Marine Fontaine (Warwick) Santiago Benavides (Warwick) |
F2F |
Parametrised landscapes and neural tube patterning (abstract) Modeling turbulent structures in the transition to turbulence (abstract) |
| 5 | 10 Feb | Yunan Yang (ETH) | F2F | Benefits of Weighted Training in Machine Learning and PDE-based Inverse Problems (abstract) |
| 6 | 17 Feb | Bindi Brook (Nottingham) | F2F | Airway remodelling in asthma - the chicken or the egg? (abstract) |
| 7 | 24 Feb | Ellen Luckins (Oxford) | F2F | Reactive decontamination of porous media (abstract) |
| 8 | 3 Mar | Carina Geldhauser (Lund) | F2F | An introduction to point vortex models (abstract) |
| 9 | 10 Mar | TBC | F2F | |
| 10 | 17 Mar | Abdessamad Belfakir (Nottingham) POSTPONED | F2F |
Term 1
| Week | Date | Speaker | Online/F2F | Title |
| 1 | 07 Oct | NO SEMINAR | ||
| 2 | 14 Oct | Philip Herbert (Heriot-Watt) | F2F |
Shape optimisation with Lipschitz functions (abstract) |
| 3 | 21 Oct | Francis Aznaran (Oxford) | F2F | Finite element methods for the Stokes–Onsager–Stefan–Maxwell equations of multicomponent flow (abstract) |
| 4 | 28 Oct | Maciej Buze (Birmingham) | F2F | Mathematical analysis of atomistic fracture and related phenomena in crystalline materials (abstract) |
| 5 | 04 Nov |
Christian Vaquero-Stainer (Warwick) Emma Davis (Warwick) |
F2F |
The sedimentation dynamics of thin, rigid disks (abstract) Using compartmental ODE models to forecast the elimination of macro-parasitic diseases (abstract) |
| 6 | 11 Nov | Fabian Spill (Birmingham) - POSTPONED | F2F | Mechanics, Geometry and Topology of Health and Disease (abstract) |
| 7 |
18 Nov |
Anna Song (Imperial & Francis Crick Institute) | Online | Describing tubular shapes and branching membranes with geometry and topology (abstract) |
| 8 | 25 Nov | Eric Neiva (Collège de France & CNRS) | F2F | Unfitted finite element methods: decoupling the mesh from the geometry (abstract) |
| 9 | 02 Dec | Catherine Kamal (Cambridge) | F2F | The microhydrodynamics of ultra-thin nanoparticles: modelling to predict the "unseen" (abstract) |
| 10 | 09 Dec | Tiina Roose (Southampton) | Online | Multiscale image based modelling of plant soil interaction (abstract) |
Abstracts
Term 3
Week 1. Abdessamad Belfakir (Nottingham) - Estimating and Predicting Dynamics of Quantum Systems Using Probability Distribution Function and Klauder Coherent States
In this talk, we present an alternative method for estimating and predicting the dynamics of quantum systems through the estimation of the probability distribution function that describes the associated density operator in the Liouville-von Neumann equation. We demonstrate the effectiveness of this approach by comparing exact and estimated solutions for a Morse oscillator interacting with an external electric field.
The second part of the talk will focus on a generalized su(2) algebra that perfectly describes the discrete energy part of the Morse potential. We also demonstrate the construction of the Klauder coherent state for Morse potential, which satisfies the resolution of identity with a positive measure obtained through the solution of the truncated Stieltjes moment problem. Finally, the time evolution of the uncertainty relation of the constructed coherent states will be analysed.
Week 2. Hanne Kekkonen (TU Delft) - Random Tree Besov Priors
Gaussian process priors are often used in practice due to their fast computational properties. The smoothness of the resulting estimates, however, is not well suited for modelling functions with sharp changes. We propose a new prior that has same kind of good edge-preserving properties than total variation or Mumford-Shah but correspond to a well-defined infinite dimensional random variable. This is done by introducing a new random variable T that takes values in the space of ‘trees’, and which is chosen so that the realisations have jumps only on a small set.
Week 3. Luis Espath (Nottingham) - A continuum framework for phase field with bulk-surface dynamics
This continuum mechanical theory aims at detailing the underlying rational mechanics of bulk-surface systems undergoing a phase transition. As a byproduct, we generalize these theories. These types of dynamic boundary conditions are described by the coupling between the bulk and surface partial differential equations for phase fields. Our point of departure within this continuum framework is the principle of virtual powers postulated on an arbitrary part where the boundary may lose smoothness. That is, the normal field may be discontinuous along an edge. However, the edges characterizing the discontinuity of the normal field are considered smooth. Our results may be summarized as follows. We provide a generalized version of the principle of virtual powers for the bulk-surface coupling along with a generalized version of the partwise free-energy imbalance. Next, we derive the explicit form of the surface and edge microtractions along with the field equations for the bulk and surface phase fields. The final set of field equations somewhat resembles the Cahn–Hilliard equation for both the bulk and surface. Moreover, we provide a suitable set of constitutive relations and thermodynamically consistent boundary conditions. We endow the bulk-surface system with two types of Robin boundary conditions. Lastly, we derive the Lyapunov-decay relations for these mixed types of boundary conditions for both the microstructure and chemical potential.
Espath, L., 2023. A continuum framework for phase field with bulk-surface dynamics. Partial Differential Equations and Applications, 4(1), p.1.
Week 4. Rachel Bennett (Bristol) - Analytical framework for coordination of metachronal cilia
On surfaces with many cilia, individual cilia coordinate their beat cycles in the form of metachronal waves. The coordinated beating facilitates self-propulsion of ciliated microorganisms and creates efficient fluid flow, which is important in several human organs. Here, we consider the connection between single cilium characteristics and the collective behaviour. A theoretical framework is presented using an array of model cilia coordinated by hydrodynamic interactions. We calculate the dispersion relation for metachronal waves and perform a linear stability analysis to identify stable waves. This framework shows how the wave vector, frequency and stability depend on the geometric properties of cilia in the array and the beat pattern of an individual cilium. These results show how information about individual cilia can be used to predict the collective behaviour of many cilia.
Week 5. Thomas Barker (Cardiff) - Continuum modelling of granular flow with dynamic compressibility
Granular flows exhibit a strong dependence on the solids volume fraction in addition to the deformation rate. In the steady inertial regime these dependencies lead to the μ(I),Φ(I)-rheology. Here I first show how this model captures key features of the steady flow of grains in vertical pipes - despite this being a complex inhomogenous flow crossing regimes. I then highlight the major shortcoming of the I-Phi paradigm: ill-posedness in transient flow at high packing fractions. Thankfully, this obstacle may be overcome by including the correct dependence on the rate of compression/dilation, which is only nonzero for transient flows.
Week 6. Jingbang Liu & Ioana Bouros (Warwick)
Modelling rare rupture of nanoscale liquid thin films - Jingbang Liu
Controlling the spontaneous rupture of nanoscale liquid thin films plays a crucial role in various applications such as thin-film solar cell manufacturing, insulation layer coating, and in lab-on-a-chip devices. Over the past few decades, theoretical work based on the long-wave theory of thin liquid films has successfully identified a critical film height, below which the surface nanowaves become linearly unstable, leading to spontaneous rupture. This dewetting in the so-called ‘spinodal regime’ has been repeatedly confirmed in experiments using atomic force microscopy on polymer films. However, rupture events are also observed for films thicker than the critical film height, which are considered linearly stable, in a different manner. It is believed that the random (Brownian) movement of particles is the cause of dewetting in this ‘thermal regime’ but the theoretical framework predicting the rupture is missing.
In this talk, we present a theory to account for the rupture of a two dimensional linearly stable thin film by utilizing fluctuating hydrodynamics and rare events theory. By modelling the film dynamics with the stochastic thin-film equation (STF) and solving it numerically, we observe rupture in the linearly stable thermal regime and record the average waiting time for rupture. We show that the STF can be rearranged into the form of a gradient flow, which allows us to apply Kramer’s law from the rare events theory to obtain a theoretical prediction of the average waiting time. Molecular dynamics (MD) simulations are also performed and we find good agreements between the numerics, the prediction, and the MD. As the average waiting time increases exponentially with the thickness of the film, adaptive multilevel splitting method is used in the simulations.
Warwick-Lancaster global COVID-19 model - Ioana Bouros
As the world recovers from the acute phase of the Covid-19 pandemic, a key issue is how to avert future large waves of hospitalisations and deaths driven by novel SARS-CoV-2 variants. Vaccination booster campaigns play an important role in maintaining the level of immunity in the population and reducing the risk of severe outcomes in infected individuals.
In this talk, we present research commissioned by the WHO SAGE Covid-19 Working Group. We consider a wave of infections caused by a novel SARS-CoV-2 variant, and investigate the effects of deploying booster vaccines according to six different age-based prioritisation strategies. We use eight different exemplar countries from around the world as test cases, chosen to cover a range of socioeconomic backgrounds. The aim is to identify which vaccine targeting strategies are most beneficial in terms of reducing infection and severe outcomes of infection, given a limited number of available booster vaccines.
This work extends earlier research conducted when the level of both infection- and vaccination-derived immunity was lower than it is now. In all countries considered, we show that prioritising the eldest and most vulnerable individuals for booster vaccination first is expected to lead to the best public health outcomes (e.g. fewest deaths). Assuming sufficient vaccine supply, serology-informed booster vaccination strategies are predicted to be of limited benefit compared to simply vaccinating the most vulnerable individuals in the population. We hope that this research is useful for guiding booster vaccination strategies in countries worldwide.
Week 7. Masha Dvoriashyna (Oxford) - Bacterial hydrodynamics: reorientation during tumbles and viscoelastic lift
Bacteria represent the major component of the world’s biomass. A number of these bacteria are motile and swim with the use of flagellar filaments, which are slender helical appendages attached to a cell body by a flexible hook. Low Reynolds number hydrodynamics is the key for flagella to generate propulsion at a microscale [1]. In this talk I will discuss two topics related to swimming of a model bacterium Escherichia coli (E. coli).
E. coli has many flagellar filaments that are wrapped in a bundle and rotate in a counterclockwise fashion (if viewed from behind the cell) during the so-called ‘runs’, wherein the cell moves steadily forward. In between runs, the cell undergoes quick ‘tumble’ events, during which at least one flagellum reverses its rotation direction and separates from the bundle, resulting in erratic motion in place. Alternating between runs and tumbles allows cells to sample space by stochastically changing their propulsion direction after each tumble. In the first part of the talk, I will discuss how cells reorient during tumble and the mechanical forces at play and show the predominant role of hydrodynamics in setting the reorientation angle [2].
In the second part, I will talk about hydrodynamics of bacteria near walls in visco-elastic fluids. Flagellar motility next to surfaces in such fluids is crucial for bacterial transport and biofilm formation. In Newtonian fluids, bacteria are known to accumulate near walls where they swim in circles [3,4], while experimental results from our collaborators at the Wu Lab (Chinese University of Hong Kong) show that in polymeric liquids this accumulation is significantly reduced. We use a combination of analytical and numerical models to propose that this reduction is due to a viscoelastic lift directed away from the plane wall induced by flagellar rotation. This viscoelastic lift force weakens hydrodynamic interaction between flagellated swimmers and nearby surfaces, which results in a decrease in surface accumulation for the cells.
References
[1] Lauga, Eric. "Bacterial hydrodynamics." Annual Review of Fluid Mechanics 48 (2016): 105-130.
[2] Dvoriashyna, Mariia, and Eric Lauga. "Hydrodynamics and direction change of tumbling bacteria." Plos one 16.7 (2021): e0254551.
[3] Berke, Allison P., et al. "Hydrodynamic attraction of swimming microorganisms by surfaces." Physical Review Letters 101.3 (2008): 038102.
[4] Lauga, Eric, et al. "Swimming in circles: motion of bacteria near solid boundaries." Biophysical journal 90.2 (2006): 400-412.
Week 8. Edward Hinton (Melbourne) - Containment of viscous fluid atop a crystal bed, and application to magmatic ore deposits
Increasing electrification of transport and energy-storage systems across the world is driving rapid growth in the consumption of key metals. Locating high-grade ores of such metals requires a detailed understanding of the physical and geological processes that governed their formation. Within flowing magma, nickel, copper, and platinum group elements are preferentially transferred into the sulphide liquid phase, which eventually forms the valuable ores. Here, we investigate the dynamics of a thin, coalesced volume of sulphide-rich liquid atop a poorly consolidated silicate melt-crystal mush. Four stages of the motion are identified with the crystal levees enabling the eventual trapping of a significant fraction of the liquid sulphide.
Week 9. Susanne Horn (Coventry) - The Elbert Range of Turbulent Rotating Magnetoconvection
Turbulent rotating magnetoconvection is fundamental to the fluid processes occurring deep inside planets, including the generation of the geodynamo in Earth’s liquid metal core. But planetary interiors are shielded from direct observation, thus, idealised, physics-driven models are essential for gaining a detailed understanding. The canonical system is Rayleigh-Benard convection, i.e. a fluid layer heated from below and cooled from above, rotated around the vertical axis and subject to an external magnetic field. This system is characterised by rich multimodal flow behaviours. Depending on the control parameters, a mix of boundary-attached, oscillatory, and geostrophic, magnetostrophic, and magnetic stationary modes constitutes the dynamics of the system. In particular, when thermal convection is subject to both a strong magnetic field and rapid rotation, two very distinct stationary modes can co-exist: a small-scale geostrophic and a large-scale magnetostrophic mode. This is in stark contrast to the monomodal stationary solution if only one of these constraints is present. The original discovery of this peculiar and unique property goes back to Donna DeEtte Elbert [1]. Recently, we expanded on the linear stability work by Elbert and derived novel predictions for the regimes at which the various types of rotating magnetoconvection occur [2]. The geophysically most relevant regime, i.e. where most planetary bodies reside, exhibits the strongest multimodality, and we coined this regime the Elbert range.
In this talk, I will address the question which of Elbert’s different modes exist and, if any, dominate in strongly nonlinear, turbulent laboratory, as well as planetary and astrophysical settings. I will present results from direct numerical simulations (DNS) of turbulent thermal rotating magnetoconvection. Examplary flow fields are shown in figure 1. Specifically, I will discuss which onset characteristics, such as the length scales and flow morphology, carry over to higher supercriticalities. I will focus on the Elbert range and explore if and how magnetostophic convection can create large length scales and thus provide favourable conditions for the dynamo generation in planetary cores.
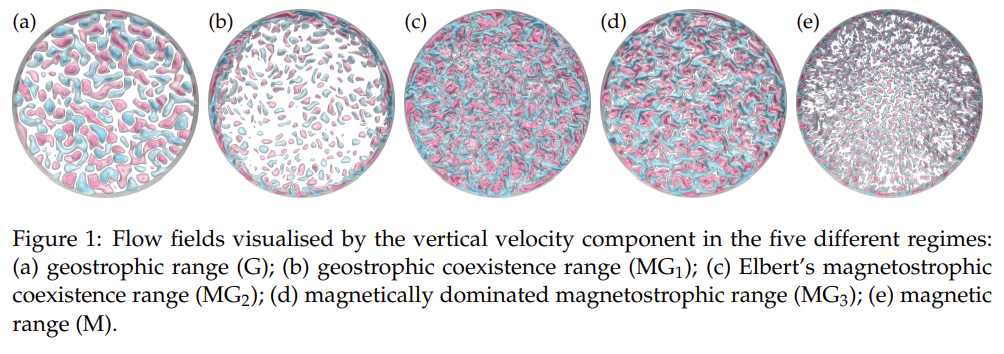
[1] Chandrasekhar, Hydrodynamic and hydromagnetic stability, Clarendon (1961)
[2] Horn and Aurnou, Proc. R. Soc. A 478, 2264 (2022)
Week 10. Eric Rogers (Southampton) - Optimization-based Iterative Learning Control with Applications in Physics, Engineering, and Healthcare
Many physical systems complete the same finite-duration task over and over again. One example in robotic applications is the `pick and place’ task, where the mission is to move a sequence of payloads from a fixed location and place them in synchronization on a moving conveyor. The series of operations is as follows: i) collect the payload from the specified location, ii) transfer it over a finite duration, iii) place it on the moving conveyor, iv) return to the starting location, and repeat this sequence for as many payloads as required or until a stop is required for maintenance or other reasons.
Iterative learning control emerged in the mid-1980s for application to examples such as the one just described, and since then has been a very active research and applications area. This seminar will first describe the development of an optimization-based design in a Hilbert space setting and then demonstrate its application, with supporting experimental results, to free-electron lasers, rack feeders, and robotic-assisted stroke rehabilitation. Some currently open research problems will also be briefly discussed.
Term 2
Week 1. Fabian Spill (Birmingham) - Mechanics, Geometry and Topology of Health and Disease
Experimental biologists traditionally study biological functions as well as diseases mostly through their abnormal molecular or cellular features. For example, they investigate genetic abnormalities in cancer, hormonal imbalances in diabetes, or an aberrant immune system in vascular diseases. However, many diseases also have a mechanical component which is critical to their deadliness. Notably, cancer kills mostly through metastasis, where the cancer cells acquire the capability to change their physical attachments and migrate. Such mechanical alterations also change geometrical features, such as the cell shape, or topological features, such as the organisation of vascular networks and cellular neighbourhoods within a tissue.
While some of these mechanical, geometrical or topological features in biology are long known, the traditional perspective is to consider them as emergent from molecular features. However, mechanical, geometrical and topological features can also affect the molecular state of a cell. Therefore, the most complete view of many biological systems is to consider them as a complex mechano-chemical systems. Diseases such as cancer are then interpreted as perturbations to this system that cannot be solely explained by considering one feature in isolation (such as a single mutation that ‘causes’ cancer).
I will discuss several examples of systems where this mechanical/geometrical/topological coupling to molecular features plays a crucial role: cells that change their shape, blood vessel cells that open gaps to let cancer cells pass during metastasis, and mitochondria that change their organisation in diabetes.
Week 2. Bryn Davies (Imperial) - Are quasicrystals the future of metamaterial waveguides?
Characterising the spectra of quasiperiodic patterns is a challenging problem that has attracted the attention of mathematicians for several decades. Metamaterial waveguides achieve spectacular wave control feats through carefully designed geometric patterns. In most applications, these patterns are typically periodic, meaning their spectra can be characterised concisely using Floquet-Bloch techniques. However, modern techniques for describing quasiperiodic patterns are now sufficiently developed that we can use them when designing metamaterial waveguides for specific applications, thereby greatly enlarging the potential design space. This talk will summarise some recent breakthroughs in the characterisation of spectral gaps in quasiperiodic metamaterials and identify opportunities where this theory can be used in applications. In particular, we will look at applications of quasicrystals to graded energy harvesting devices and symmetry-induced waveguides.
Week 3. Graham Benham (Oxford) - From Olympic rowing to gunwale bobbing: Wave drag and wave thrust phenomena
In this talk I will discuss different wave phenomena associated with motion on the surface of water. I’ll start with some previous work on waves in Olympic rowing and how to reduce drag, either by tuning the shape of the boat, or by exploiting hysteresis patterns in regions of shallow water. Then I’ll talk about current research within the theme of wave-driven propulsion, i.e. how thrust can be generated from surfing the gradients of a self-generated wave-field. This phenomenon occurs across a wide range of scales, from tiny walking droplets in a vibrating bath, to jumping up and down on the sides of a canoe to drive it forwards, also known as gunwale bobbing.
Week 4. Marine Fonatine & Santiago Benavides (Warwick)
Parametrised landscapes and neural tube patterning - Marine Fontaine
During the early development of the vertebrate nervous system, the elongated axis of the neural tube is divided into a number of genetically distinct domains of progenitor cells. Experiments show that this patterning depends on two different signalling pathways: the amount of signal that a cell receives allocates it to a unique domain characterised by its gene-expression. We are attempting to construct models that gather tools from singularity theory and dynamical systems to understand how cells can transition between the distinct domains when exposed to different signal regimes.
The mathematical formalism I will discuss in this talk is inspired by the Waddington metaphor in which the transitioning dynamics of a cell is conceived as a flow going down a landscape whose attractors correspond to progenitor domains. As these landscapes are signal-dependent, they come in a parametrised family. Changing signals can produce bifurcations that force the cells trapped in a domain to transition to a new fate. Although these landscapes do not model the complex dynamics of the underlying gene regulatory network, they are able to capture the relevant qualitative changes that we observe in the biological datas.
Modeling turbulent structures in the transition to turbulence - Santiago Benavides
The transition from simple laminar flow to the chaotic and multi-scale turbulent state in many wall-bounded shear flows is subcritical: the laminar flow remains linearly stable to perturbations, and the transition to turbulence occurs through the local proliferation of large-scale turbulent patches throughout the domain. The phenomenon is inherently nonlinear and involves the nontrivial interaction of turbulent and laminar regions. Despite the success of models capturing the dynamics of these turbulent structures in pipe flow (an effectively one-dimensional geometry for the patches of turbulence), the large-scale organization of turbulent structures in effectively two-dimensional planar geometries remains a challenge. In this work, we take an important step towards this direction by introducing a large-scale model which reproduces the phenomena observed in simulations and experiments in these geometries, including banded turbulent structures.

Turbulent bands reproduced from Duguet et al. JFM 650 (2010).
Week 5. Yunan Yang (ETH) - Benefits of Weighted Training in Machine Learning and PDE-based Inverse Problems
Many models in machine learning and PDE-based inverse problems exhibit intrinsic spectral properties, which have been used to explain the generalization capacity and the ill-posedness of such problems. In this talk, we discuss weighted training for computational learning and inversion with noisy data. The highlight of the proposed framework is that we allow weighting in both the parameter space and the data space. The weighting scheme encodes both a priori knowledge of the object to be learned and a strategy to weight the contribution of training data in the loss function. We demonstrate that appropriate weighting from prior knowledge can improve the generalization capability of the learned model in both machine learning and PDE-based inverse problems.
Week 6. Bindi Brook (Nottingham) - Airway remodelling in asthma - the chicken or the egg?
Inflammation, airway hyper-responsiveness (which causes constriction of the airways at lower trigger levels than in normal subject) and airway remodelling (long term structural changes of the airway wall) are key features of asthma. While this is well-established, it is not clear how they are linked or whether they are causes or symptoms of the disease. In this talk I will give an overview of the multiscale biomechanical models we have developed to understand how smooth muscle contraction at the cell level translates to tissue level airway constriction during an asthma attack. Then I will describe a long-timescale theoretical model, developed in parallel with an experimental study, that accounts for mechanochemical drivers of airway remodelling with some illustrative results. And finally I will describe some work in progress: how the combination of both experimental data and the mechanistic model might be used to understand maintenance of homoeostasis in healthy airways and therefore what perturbations might drive the airway into a diseased state.
Week 7. Ellen Luckins (Oxford) - Reactive decontamination of porous media
Following a chemical weapons attack, it is crucial for public health that the toxic chemical agent is properly cleaned up. One particular issue is when the agent has contaminated porous materials, such as brick or concrete. In such cases, decontamination is typically achieved by neutralising the agent with a cleanser in a chemical reaction. It is relatively straightforward to write down a model that describes the interplay of the agent and cleanser fluids on the scale of the pores, but very computationally expensive to solve such a model over realistic spill sizes. In this talk I will present homogenised PDE models for the reactive decontamination of porous media, which are computationally efficient to simulate while still taking the pore-scale behaviour into account. Solutions of these homogenised models show how differences in the initial distribution of agent within the pore-space affect both the decontamination time and the amount of cleanser required to fully decontaminate the porous material.
Week 8. Carina Geldhauser (Lund) - An introduction to point vortex models
In this talk we will discuss a family of discrete models for atmospheric turbulence, often called point vortex models. They have been originally derived by Helmholtz, about 130 years ago, but many interesting questions are still open.
We will show how point vortices provide an approximation of solutions to generalized surface quasi-geostrophic models, a family of fractional PDEs which interpolate between 2D Euler equations and the more irregular SQG equation.
Lastly, we will briefly touch upon how variational methods and tools from probability theory together can help us to obtain more information about turbulence phenomena. Joint work with Marco Romito (Uni Pisa).
Week 9. Susanne Horn (Coventry) - TBA
Week 10. Abdessamad Belfakir (Nottingham) - TBA
Term 1
Week 1. No Seminar
Week 2. Philip Herbert (Heriot-Watt) - Shape optimisation with Lipschitz functions
In this talk, we discuss a novel method in PDE constrained shape optimisation. We begin by introducing the concept of PDE constrained shape optimisation. While it is known that many shape optimisation problems have a solution, their approximation in a meaningful way is non-trivial. To find a minimiser, it is typical to use first order methods. The novel method we propose is to deform the shape with fields which are a direction of steepest descent in the topology of . We present an analysis of this in a discrete setting along with the existence of directions of steepest descent. Several numerical experiments will be considered which compare a classical Hilbertian approach to this novel approach.
Week 3. Francis Aznaran (Oxford) - Finite element methods for the Stokes–Onsager–Stefan–Maxwell equations of multicomponent flow
The Onsager framework for linear irreversible thermodynamics provides a thermodynamically consistent model of mass transport in a phase consisting of multiple species, via the Stefan–Maxwell equations, but a complete description of the overall transport problem necessitates also solving the momentum equations for the flow velocity of the medium. We derive a novel nonlinear variational formulation of this coupling, called the (Navier–)Stokes–Onsager–Stefan–Maxwell system, which governs molecular diffusion and convection within a non-ideal, single-phase fluid composed of multiple species, in the regime of low Reynolds number in the steady state. We propose an appropriate Picard linearisation posed in a novel Sobolev space relating to the diffusional driving forces, and prove convergence of a structure-preserving finite element discretisation. This represents some of the first rigorous numerics for the coupling of multicomponent molecular diffusion with compressible convective flow. The broad applicability of our theory is illustrated with simulations of the centrifugal separation of noble gases and the microfluidic mixing of hydrocarbons. This is joint work with Alexander Van-Brunt.
Week 4. Maciej Buze (Birmingham) - Mathematical analysis of atomistic fracture and related phenomena in crystalline materials
The modelling of atomistic fracture and related phenomena in crystalline materials poses a string of mathematically non-trivial and exciting challenges, both on the theoretical and practical level. At the heart of the problem lies a discrete domain of atoms (a lattice), which exhibits spatial inhomogeneity induced by the crack surface, particularly pronounced in the vicinity of the crack tip. Atoms interact in a highly nonlinear way, resulting in a severely non-convex energy landscape facilitating non-trivial behaviour of atoms such as (i) crack propagation; (ii) near-crack tip plasticity - emission and movement of defects known as dislocations in the vicinity of the crack tip; (iii) surface effects - atoms at the crack surface relaxing or possibly attaining an altogether different crystalline structure. On the practical side, the richness of possible phenomena renders the task of setting up numerical simulations particularly tricky - numerical artefacts, e.g. induced by prescribing a particular boundary condition, can lead to inconsistent results.
In this talk I will aim to summarise on-going efforts aimed at putting the atomistic modelling of fracture on a rigorous mathematical footing. I will introduce a framework giving rise to well-defined models for which regularity and stability of solutions can be discussed (topic of my PhD thesis at Warwick). I will then show how the theory can be used to set up practical simulations, such as Mode I fracture of silicon on the (111) cleavage plane using state-of-the-art interatomic potentials. I will also outline how this framework can be used to rigorously derive upscaled models of near-crack-tip plasticity. Finally, I will also talk about challenges in addressing the surface effects.
Week 5. Christian Vaquero-Stainer & Emma Davis (Warwick)
The sedimentation dynamics of thin, rigid disks - Christian Vaquero-Stainer
Sedimentation problems arise in a wide range of natural and industrial processes and exhibit a rich array of phenomenology. A particular motivation for this study is the size segregation of graphene flakes, for which a dominant method is centrifugation in a viscous fluid (Khan 2012). We present a numerical investigation of the sedimentation dynamics of thin, deformed circular disks sedimenting freely under gravity in an otherwise quiescent, Stokesian fluid. In the first part of this study, we address singularities which arise in the fluid pressure and velocity gradient at the edge of the disk, by developing an augmented finite element method to capture the singularities with analytic functions. In the second part of the study, we deploy this method in a fluid-structure interaction framework to examine the behaviour of two distinct classes of disk shape, namely cylindrically- and conically-deformed disks with one and two planes of symmetry, respectively. We explore the geometry-driven dynamics and the bifurcation structure which arises for the conically-deformed disk as the level of asymmetry is varied.
Using compartmental ODE models to forecast the elimination of macro-parasitic diseases - Emma Davis
Standard compartmental models of infectious disease transmission work by categorising a population by stage of infection and then building a system of differential equations that govern the density or number of individuals in each category, e.g. the SIR model has compartments for susceptible (S), infectious (I) and recovered/removed (R) individuals. This makes sense when we are interested in the number of individuals infected over an epidemic where infection is a binary state, as measured by prevalence and incidence, but is less useful for macro-parasitic diseases, where infection is instead classified by the number of macro-parasites inhabiting any given individual. Models for macro-parasitic diseases therefore more commonly consider the number of parasites per individual (their parasite “burden”) or, on a population scale, the mean parasite burden. Common biological features of macro-parasitic diseases, such as sexual reproduction of the parasites or indirect transmission routes, and aggregation between individuals, can result in interesting dynamics at low prevalence, which I will discuss using the example of the macro-parasitic disease lymphatic filariasis.
Week 6. Fabian Spill (Birmingham) - Mechanics, Geometry and Topology of Health and Disease
Experimental biologists traditionally study biological functions as well as diseases mostly through their abnormal molecular or cellular features. For example, they investigate genetic abnormalities in cancer, hormonal imbalances in diabetes, or an aberrant immune system in vascular diseases. However, many diseases also have a mechanical component which is critical to their deadliness. Notably, cancer kills mostly through metastasis, where the cancer cells acquire the capability to change their physical attachments and migrate. Such mechanical alterations also change geometrical features, such as the cell shape, or topological features, such as the organisation of vascular networks and cellular neighbourhoods within a tissue.
While some of these mechanical, geometrical or topological features in biology are long known, the traditional perspective is to consider them as emergent from molecular features. However, mechanical, geometrical and topological features can also affect the molecular state of a cell. Therefore, the most complete view of many biological systems is to consider them as a complex mechano-chemical systems. Diseases such as cancer are then interpreted as perturbations to this system that cannot be solely explained by considering one feature in isolation (such as a single mutation that ‘causes’ cancer).
I will discuss several examples of systems where this mechanical/geometrical/topological coupling to molecular features plays a crucial role: cells that change their shape, blood vessel cells that open gaps to let cancer cells pass during metastasis, and mitochondria that change their organisation in diabetes.
Week 7. Anna Song (Imperial) - Describing tubular shapes and branching membranes with geometry and topology
Tubular and membranous shapes are important mathematical structures that arise in many biomedical applications. Morphology is linked to function: their branching patterns, shaped by interactions and remodelled by diseases, inform us on a biological system.
I will present the “curvatubes” model, which unifies a wide continuum of porous shapes within a common geometric framework (https://doi.org/10.1007/s10851-021-01049-9). It generalizes the Helfrich model for biomembranes by considering shapes as optimizers of a curvature functional in which the principal curvatures may play asymmetric roles. The geometric problem is approximated by a novel phase-field formulation that satisfies a Gamma-limsup property, and is readily implementable as a GPU algorithm. The framework is very flexible and shape textures can be aligned, spatialized, or constrained on a domain.
In the remaining time, I will introduce some topological approaches to analyze such structures using persistent homology, and how they may empirically quantify the "texture of shapes". These are tested on proprietary images of bone marrow vasculature remodelled in acute myeloid leukaemia.
Overall, these compact descriptions offer a unified view to branching tubules and membranes, and will potentially lead to applications in bioengineering, imaging, or materials science.
Week 8. Eric Neiva (Collége de France & CNRS) - Unfitted finite element methods: decoupling the mesh from the geometry
The finite element method (FEM) approximates a PDE from a variational formulation of the problem. Its standard formulation requires a mesh fitting to the boundary of the geometry of interest. Yet, for many problems of practical interest, the geometry is so intricate that mesh generation requires frequent and time-consuming manual intervention. Boundary-fitted meshing can be avoided with unfitted or immersed FEMs. The main idea is to embed the geometry in a simple mesh (e.g., a Cartesian grid) and define the discretisation in the cells intersecting the geometry. In this talk, we will describe a novel unfitted FEM that circumvents the classical issue of immersed FEM: ill-conditioning due small cell-to-geometry intersections We will discuss its application to early embryo development in animals.
Week 9. Katherine Kamal (Cambridge) - The microhydrodynamics of ultra-thin nanoparticles: modelling to predict the "unseen"
Graphene nanoparticles are ubiquitous, used in everything from the design of more robust extreme weather-resistance spacecraft to flexible-electronics tracks. Made from just a few atomic layers, the instantaneous dynamics of these plate-like particles in flowing liquids are, experimentally, practically inaccessible. We study theoretically and computationally the microhydrodynamics of dilute suspensions of graphene in a simple viscous shear flow field. In the infinite Péclet number limit, a rigid platelet with the interfacial hydrodynamic slip properties of graphene does not follow the periodic rotations predicted for classical colloidal particles but aligns itself at a slight inclination angle with respect to the flow. This unexpected result is due to the hydrodynamic slip reducing the tangential stress at the graphene-liquid surface. By analysing the Fokker-Plank equation for the orientational distribution function for decreasing Péclet numbers, we explore how hydrodynamic slip affects the particle’s orientation and effective viscosity. We find that hydrodynamic slip can dramatically change the average particle’s orientation and effective viscosity. For example, the effective viscosity of a dilute suspension of graphene platelets is predicted to be smaller than the base fluids under certain flow conditions for typical slip length values.
Week 10. Tiina Roose (Southampton) - Multiscale image based modelling of plant soil interaction
We rely on soil to support the crops on which we depend. Less obviously we also rely on soil for a host of 'free services' from which we benefit. For example, soil buffers the hydrological system greatly reducing the risk of flooding after heavy rain; soil contains very large quantities of carbon, which would otherwise be released into the atmosphere where it would contribute to climate change. Given its importance it is not surprising that soil, especially its interaction with plant roots, has been a focus of many researchers. However the complex and opaque nature of soil has always made it a difficult medium to study.
In this talk I will show how we can build a state of the art image based model of the physical and chemical properties of soil and soil-root interactions, i.e., a quantitative, model of the rhizosphere based on fundamental scientific laws.
This will be realised by a combination of innovative, data rich fusion of structural and chemical imaging methods, integration of experimental efforts to both support and challenge modelling capabilities at the scale of underpinning bio-physical processes, and application of mathematically sound homogenisation/scale-up techniques to translate knowledge from rhizosphere to field scale. The specific science questions I will address with these techniques are: (1) how does the soil around the root, the rhizosphere, function and influence the soil ecosystems at multiple scales, (2) what is the role of root- soil interface micro morphology on plant nutrient uptake, (3) what is the effect of plant exuded mucilage on the soil morphology, mechanics and resulting field and ecosystem scale soil function and (4) how to translate this knowledge from the single root scale to root system, field and ecosystem scale in order to predict how the climate change, different soil management strategies and plant breeding will influence the soil fertility.
See also:
Mathematics Research Centre
Mathematical Interdisciplinary Research at Warwick (MIR@W)
Past Events
Past Symposia
Where possible, visitors should obtain an EDUROAM account from their own university to enable internet access whilst at Warwick.
You can register for any of the symposia or workshops online. To see which registrations are currently open and to submit a registration, please click hereLink opens in a new window.
Mathematics Research Centre
Zeeman Building
University of Warwick
Coventry CV4 7AL - UK
E-mail:
MRC@warwick.ac.uk

