Katarzyna Macieszczak

Experimental realisations of quantum systems can be considered isolated or closed only on the timescales when the influence of their environments can be neglected. At later times, interactions with the environments introduce external noise – decoherence – leading to mixed rather than pure system states. Asymptotically, any information about initial conditions is usually fully erased and such open quantum systems are described by unique stationary states. Since those are typically found far from equilibrium, either due to the presence of the Hamiltonian drive or because of dissipative environments, they can no longer be determined in terms of thermodynamic ensembles. With only few cases solvable analytically, they need to be investigated numerically instead, which limits their study to moderate system sizes.

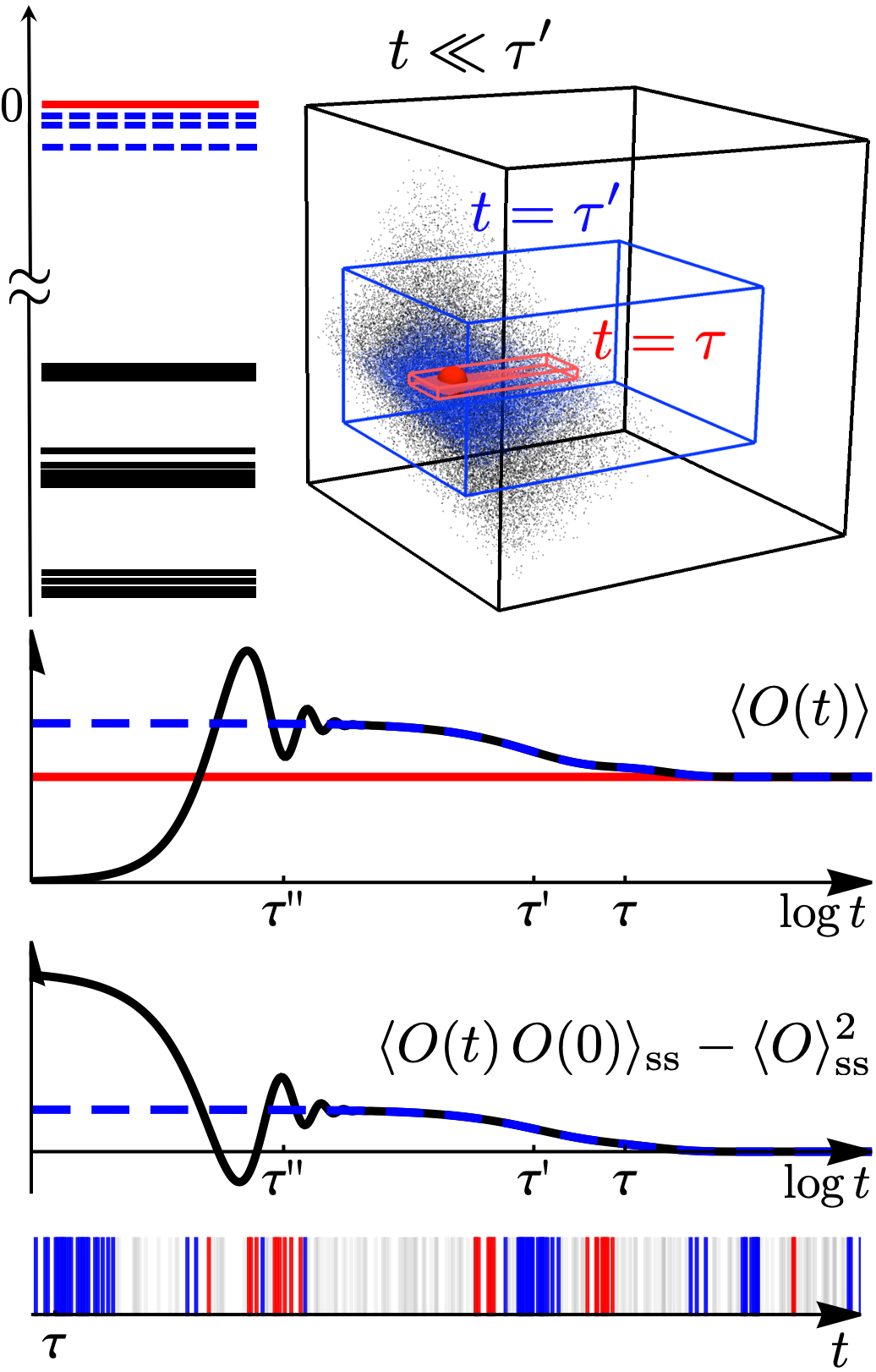
The process of relaxation towards a stationary state can be very complex, especially in many-body systems. Multiple timescales may arise in the dynamics, leading to the existence of time regimes during which system states appear to be stationary despite their significant difference from the true stationary state. This phenomenon of metastability is well understood for classical equilibrium systems – as a consequence of multiple local minima present in free energy functionals – but remains largely unexplored for non-equilibrium open quantum systems, where a general connection between dynamical and static properties is elusive. In the context of quantum statistical physics, its importance extends to understanding stationary state transitions – occurring in the thermodynamic limit of infinite system size that cannot be simulated – as they give rise to metastability of large enough systems, so that its study can be used to uncover non-equilibrium phase diagrams.

Crucially, metastability enables a part of the information initially encoded in the system to survive over an extended period of time virtually unaffected by the noise. For open quantum systems this may correspond to both classical and quantum long-lived characteristics, with the latter ensuring the possibility of robust quantum coherences or even robust quantum entanglement – resources necessary for quantum technology applications. It remains an open question how to identify, and then to control such properties, not only in numerical simulations but especially for experimental settings where only limited operations are available.

See Phys. Rev. Research 3, 033047 (2021).